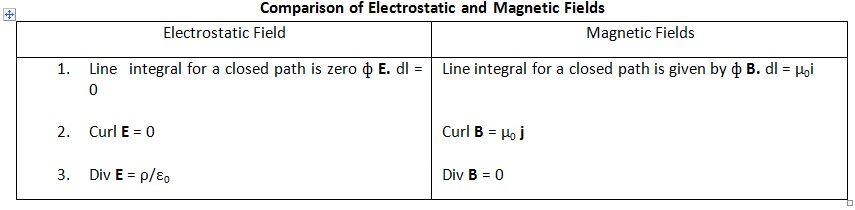
Differential Form of Amperes Law

Let J be the current density in an element dS of the surface bounded by the closed path. Then,
total current i = ∫_s J. dS
. :     φ B. dl = μ₀ ∫_s  j. dS
Using strokes theorem, φ B. dl = μ₀ ∫_s curl B.dS
. :    ∫_s curl B.dS = μ₀ ∫_s  j. dS
Or     curl B = μ₀ j
This is the differential form of Ampere’s law.

Divergence of Magnetic Field Vector B

Divergence of a magnetic field vector B is defined as the flux through a surface S enclosing a unit volume.
        Div B = Flux/v = ∫_s B. dS / v
We know that magnetic lines of induction are closed curves. If we construct any closed surface must also leave it. In other words, the net in flux is equal to the net efflux of lines of force. Thus the total normal magnetic flux over a closed surface is zero.
        ∫_s B. dS  = 0
or div B = 0 (every where)



For more help in Differential Form of Amperes Law click the button below to submit your homework assignment